About Mirrors and Symmetry
There are a number of active/passive patterns which look like they are either a mirror or a reverse of another pattern. For instance, the patterns for Ruleset 020 (on the left) and Ruleset 006 appear to be mirrors of each other:
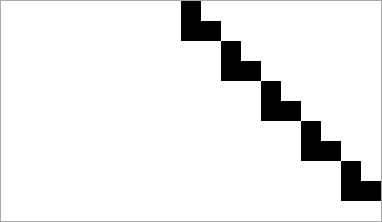

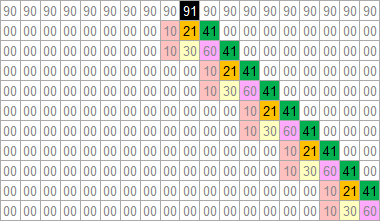
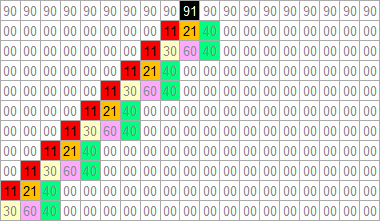
Examining rules used, however, shows another picture. The rules invoked by the two rulesets result in two patterns which do not mirror each other:
A similar finding holds for symmetrical active/passive patterns. Below are the active/passive and rules used patterns for Ruleset 018. Symmetry in the active/passive pattern is subverted by the mechanics of the rules used:

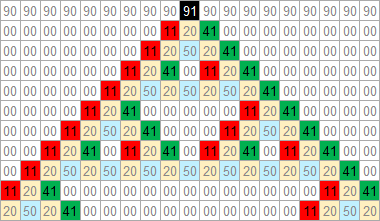
I haven't poked further at this observation, particularlly as it may affect the mechanics of automata, but clearly handedness (chirality) is at play. It is fair to say that looking at rules used adds another level understanding to the study of automata complexity.
Within the community of researchers who study active/passive patterns, it is generally accepted that, after removing mirrors and reverses, the 256 rulesets generate only 88 unique patterns. My quick calculation suggests there are 144 unique patterns when rules used are taken into consideration.
Home
Prev: Beginnings & the Use of Color
Next: The First Row and Grouping Results